Category Archives: computer science
Computing with SciPy
Transform Science - Enthought
The One Programming Language to Rule Them All
There’s a language that sits a layer beneath code, and it yearns to be explored
At the time of writing, “which programming language should I learn” yields 517 million search results. Each page will go on about the advantages one language has over the others, and 90% of them will end up recommending Python or JavaScript.
If I may be so bold, I would like to formally disagree with all 517 million of these results and suggest that the first programming language you should learn is logic.
Knowing how to code just doesn’t cut it anymore. The market is so saturated with bootcamp graduates that the “junior software developer” position has been wiped from existence. To succeed today, you need to know how to code and have a logical mindset to boot.
My First Computer Science Lesson
My first exposure to Computer Science was an elective I took in 10th grade. On day one, I was elated to see a wide spread of ice cream and a variety of sundae toppings before me. After we all took our seats, my teacher proclaimed:
“Today, we’re going to be making sundaes. Under one condition: you have to write a list of specific instructions on how to prepare your sundae — then I’ll follow them.”
No problem, I thought, this will be a breeze. In under a minute, I jotted down the perfect set of sundae-making instructions:
Scoop three scoops of black raspberry ice cream into a bowl Pour two tablespoons of hot fudge into said bowl Put whipped cream into the bowl Place sprinkles and a cherry on top of the sundae
Then my teacher — the computer in this lovely metaphor — put on the most accurately sarcastic display I’ve ever seen. She started viciously stabbing the ice cream carton, lid intact, unable to penetrate its tough exterior.
“OK, remove the lid first,” I said, desperate for a treat.
“You failed to provide me with those instructions, so, unfortunately, I failed to make you a sundae, NEXT!”
Fast-forward to attempt #2
Open the black raspberry ice cream by removing the lid Scoop three scoops of black raspberry ice cream into a bowl Open the hot fudge and pour two tablespoons into the bowl Open the whipped cream and add some to the bowl Place sprinkles and a cherry on top of the sundae
This time I was sure I had it. I even went ahead and ensured each item was opened before adding it to my masterpiece.
She opened the lid, scooped three scoops, and put them into the bowl. At last, my nascent sundae was finally coming into fruition. Then she opened the hot fudge and placed two tablespoons into my bowl. Not two tablespoons of hot fudge, mind you — two actual spoons, and no hot fudge. I failed to be specific enough — again. When all was said and done, I was handed a bowl of ice cream laden with two metal spoons, a solid canister of whipped cream, and about 300 sprinkles.
I think by this point it finally clicked: the computer is a purely logical entity. It has no context and makes no assumptions. It responds to a very specific set of instructions and follows them to a T.
My final set of sundae-making instructions was a verbose, but necessary, disaster:
If they are not already, open each of the following: Black Raspberry Ice Cream, Hot Fudge, Sprinkles, and Whipped Cream Aquire a bowl from the stack and place it in front of you Grab the ice cream scoop and, one at a time, scoop three scoops of black raspberry ice cream into the bowl. Place the scoop down when done Aquire the hot fudge spoon if not already in your possession, then fetch two tablespoons of hot fudge and place them into the bowl, one at a time, and put down the hot fudge when done Turn the whipped cream upside down, press your finger against the nozzle over the bowl for 3 seconds, and return the bottle to its resting position Sprinkle approximately 40 sprinkles over the bowl and return the shaker to its upright position when done Fetch a single cherry from the cherry jar and place it delicately on top of the sundae Hand the sundae to the student along with a spoon
That last bullet was extremely important because she started eating my sundae without it.
This is the reality of computer programming. Providing intense sets of detailed instructions to a computer. In essence, this is what all programming languages decompose into — instructions.
The Software Development Career Path
Software development is now at a point where it’s too broad to discuss as a single industry, just as “software developer” is too broad a job title. Two developers can be equally marketable while having disjointed skill sets, implying there is more to a career in development than the mere ability to code. There’s an attribute skilled developers have that is universal and separate from programming — logic.
The best developers are experts in critical thinking. This is essential because the majority of software projects are undocumented, splintered disasters. They require a critical thinker to piece information together and fill in the gaps when needed. The developers who lag behind are those who cannot connect the dots.
All this culminates to another bold statement, this time in bold: The fundamentals of computer science are, and will always be, paramount to coding ability.
Popular languages come and go with the tide. Frameworks become deprecated, and companies react to shifting demands by mixing up their tech stack. The one thing that never changes? Fundamentals — that’s literally their definition!
How to Improve Logical Thinking
For those who can’t quite trek a mountain for deep thought, consider these tools to improve your programmatic critical thinking:
Know your run time complexities
Also referred to as Big-O, the runtime complexity of a program can be expressed as the number of steps taken on any instance in relation to the size of the input (n). Keeping perpetual tabs on the runtime of your programs is step one.
Know your data structures
Data structures are at the heart of every complex program. Knowing which structure to use in what scenario is an art of its own. Data structures tie directly into runtime complexities, as picking the wrong structure can send your programs to a grinding halt. Searching an Array for a value is O(n), which means it gets more expensive to use Arrays as the size of your input increases. Hash lookups are O(1), so the lookup time for a Hash key will be constant, regardless of the number of keys in said Hash.
I’ve had candidates argue that an Array has a faster search time than a Hash. This was an immediate signal not to hire them — know your data structures.
Read/watch/listen
Sites like Udemy, Pluralsight, and Codecademy are incredible resources for learning new programming languages. For fundamentals, turn to books on general engineering concepts, best practices, and coding styles. The most highly recommended books for engineers are Design Patterns, Refactoring, Code Complete, Clean Code, and The Pragmatic Programmer, to name a few. Lastly, every engineer should keep a copy of “Introduction to Algorithms” in their desk for safe keeping.
Practice!
You cannot become a master violinist without excessively playing the violin. Sites like HackerRank, CodeWars, CoderByte, TopCoder, and LeetCodehave thousands of challenge problems designed to test your knowledge of data structures and algorithms. The best approach I’ve found to using these sites is to take your own shot at solving the problem, host your solutions on Github, and then look at the top solutions for that problem to see how others approached it. Which brings me to my last point:
View others’ code
The greatest mistake you can make in your software development journey is to go it alone. Software development is a largely crowdsourced effort. We build standards together, make mistakes together, and learn what works over time (by failing a lot). Taking the time to read skilled developers’ code will always pay off. Just make sure it’s good code.
The best advice I can leave you with is to never feel ashamed of what you don’t (yet) know. As I mentioned, this industry is massive, its number of languages extreme, and the content dense. It takes a great deal of time and effort to build an understanding, even more to gain proficiency, and an immense amount more to gain mastery. I’ll let you know when I get there.
Better Programming
Advice for programmers.
Applause from you and 2,671 others

WRITTEN BY
Boston Software Engineer specializing in Hadoop and big-data development. I enjoy writing about that which I’m passionate about.
Origame Code
The Elements of Computing Systems
Stack Overflow: The Architecture - 2016 Edition
What would you do with $700?
CS and Obama
Symbol Table - assembler
360 virtual reality
Online Programming Visualizer
The New Boston
027 - Example 01 - Coin Flip - Part 01 (Pseudocode)
13. Breadth-First Search (BFS)
Taylor Models
zetcode
ZetCode brings tutorials for programmers in various areas. The main are Graphical User Interfaces, databases, and programming languages. The website's mission is to provide competent, quick and easy to understand tutorials for modern-day technologies.
News
PostgreSQL Ruby tutorial written (August 2, 2015)
PostgreSQL C tutorial written (July 30, 2015)
GTK+ 2 tutorial cleaned and updated (July 20, 2015)
JavaFX tutorial written (June 26, 2015)
Java SWT tutorial cleaned and updated (June 5, 2015)
Advanced Java Swing e-book published (May 23, 2015)
Windows API cleaned and updated (March 26, 2015)
PyQt5 tutorial written (January 28, 2015)
Tcl tutorial cleaned and updated (January 25, 2015)
SQLite tutorial updated (November 18, 2014)
SQLite C tutorial written (November 12, 2014)
Coming
Arduino, JavaScript tutorial, Mono tutorial, PyGame tutorial, CSS tutorial, C tutorial, C++ tutorial, Firebird tutorial, PostgreSQL tutorial, JQuery tutorial, Python 3 tutorial, D tutorial, Apache Tomcat tutorial, Gambas tutorial, Java Games e-book, Advanced Qt e-book, Flex tutorial, Java Servlets tutorial, Java IO tutorial, LINQ tutorial, Ant tutorial, SQLAlchemy tutorial, AWK tutorial, Bash tutorial
Comp Sci at Google's New Portal
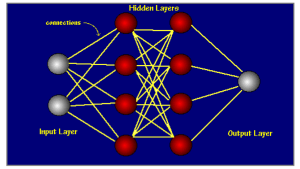
A Neural Network in 11 lines of pyhton
Comp Sci MS online
Simulation Driven Engineering
Model Based Design
Minecraft Shows Robots How to Stop Dithering
Javafx tutorial
Game Programming with Java
What is code?
Will's gitHub
Principles of computing part 1 - coursera
2048 game at coursera
WeMips
Codepad
Coding helpers

Ladyada: Ask an engineer
LWJGL Tutorial Series
StdIn.java notes
public static void main(String[] args) {
final double G = 6.67 * Math.pow(10,-11);
final double T = Double.parseDouble(args[0]);
final double DELTAT = Double.parseDouble(args[1]);
String tablePlanet = "mercury";
int numPlanets = Integer.parseInt(StdIn.readString());
double universeRadius = Double.parseDouble(StdIn.readString());
Solar system - phet
OpenGL Advanced Rendering (VBO, DL, VA) - LWJGL Tutorials
How to install LWJGL
HSCTF
The Coding Universe
Cryptography Exercises
10 Codes and Ciphers
Apophysis at DEviantart
Curves and surfaces for computer graphics
Programmer's stackexchange
Minecraft Unblock for Education
TRAER.PHYSICS 3.0
Case Study: N-body Simulation
N-body Simulations - Code
N-body Simulations - Code Part 1
Object-oriented programming
In general, programming languages have built in objects, such as integers, float point numbers, booleans, arrays, etc. that can be used very easily by the user. These allow programmers to code virtually whatever they need, but it is often easier to create objects that better suit the application that is being coded. The following examples are written in Java, so a small background would be useful. Even though the syntax may be slightly different, other programming languages such as C++ and Python can accomplish identical tasks.
It would be nice to declare a body, b, with the following parameters:
Body b = new Body(double rx, double ry, double vx, double vy, double mass, Color color)
This is accomplished, in Java, using the code below. Comments are prefaced by //.
import java.awt.Color;
public class Body {
private static final double G = 6.673e-11; // gravitational constant
private static final double solarmass=1.98892e30;
public double rx, ry; // holds the cartesian positions
public double vx, vy; // velocity components
public double fx, fy; // force components
public double mass; // mass
public Color color; // color (for fun)
// create and initialize a new Body
public Body(double rx, double ry, double vx, double vy, double mass, Color color) {
this.rx = rx;
this.ry = ry;
this.vx = vx;
this.vy = vy;
this.mass = mass;
this.color = color;
}
// update the velocity and position using a timestep dt
public void update(double dt) {
vx += dt * fx / mass;
vy += dt * fy / mass;
rx += dt * vx;
ry += dt * vy;
}
// returns the distance between two bodies
public double distanceTo(Body b) {
double dx = rx - b.rx;
double dy = ry - b.ry;
return Math.sqrt(dx*dx + dy*dy);
}
// set the force to 0 for the next iteration
public void resetForce() {
fx = 0.0;
fy = 0.0;
}
// compute the net force acting between the body a and b, and
// add to the net force acting on a
public void addForce(Body b) {
Body a = this;
double EPS = 3E4; // softening parameter (just to avoid infinities)
double dx = b.rx - a.rx;
double dy = b.ry - a.ry;
double dist = Math.sqrt(dx*dx + dy*dy);
double F = (G * a.mass * b.mass) / (dist*dist + EPS*EPS);
a.fx += F * dx / dist;
a.fy += F * dy / dist;
}
// convert to string representation formatted nicely
public String toString() {
return "" + rx + ", "+ ry+ ", "+ vx+ ", "+ vy+ ", "+ mass;
}
}
This defines the Body object that we will use to store information about bodies in our simulation. This file would be saved in a file Body.java and compiled into a class Body.class. The class file should reside in the same directory as all other class files.
On the next page we will create the main class that uses these methods to run the simulation.
N-body Simulations- Code Part 2
Using our Body object
Recall that by creating a new class, we have a Body object that can be called using:
Body b = new Body(double rx, double ry, double vx, double vy, double mass, Color color)
Now, we want to use the brute-force method to update the positions and velocities of the bodies. Here's the code for an applet that we'll see at the end of this tutorial.
BruteForce.java
// tell the compiler where to find the methods you will use.
// required when you create an applet
import java.applet.*;
// required to paint on screen
import java.awt.*;
import java.awt.event.*;
//Start the applet and define a few necessary variables
public class BruteForce extends Applet {
public int N = 100;
public Body bodies[]= new Body[10000];
public TextField t1;
public Label l1;
public Button b1;
public Button b2;
public boolean shouldrun=false;
// The first time we call the applet, this function will start
public void init()
{
startthebodies(N);
t1=new TextField("100",5);
b2=new Button("Restart");
b1=new Button("Stop");
l1=new Label("Number of bodies:");
ButtonListener myButtonListener = new ButtonListener();
b1.addActionListener(myButtonListener);
b2.addActionListener(myButtonListener);
add(l1);
add(t1);
add(b2);
add(b1);
}
// This method gets called when the applet is terminated. It stops the code
public void stop()
{
shouldrun=false;
}
//Called by the applet initally. It can be executed again by calling repaint();
public void paint(Graphics g)
{
g.translate(250,250); //Originally the origin is in the top right. Put it in its normal place
//check if we stopped the applet, and if not, draw the particles where they are
if (shouldrun) {
for(int i=0; i<N; i++) {
g.setColor(bodies[i].color);
g.fillOval((int) Math.round(bodies[i].rx*250/1e18),(int) Math.round(bodies[i].ry*250/1e18),8,8);
}
//go through the Brute Force algorithm (see the function below)
addforces(N);
//go through the same process again until applet is stopped
repaint();
}
}
//the bodies are initialized in circular orbits around the central mass.
//This is just some physics to do that
public static double circlev(double rx, double ry) {
double solarmass=1.98892e30;
double r2=Math.sqrt(rx*rx+ry*ry);
double numerator=(6.67e-11)*1e6*solarmass;
return Math.sqrt(numerator/r2);
}
//Initialize N bodies with random positions and circular velocities
public void startthebodies(int N) {
double radius = 1e18; // radius of universe
double solarmass=1.98892e30;
for (int i = 0; i < N; i++) {
double px = 1e18*exp(-1.8)*(.5-Math.random());
double py = 1e18*exp(-1.8)*(.5-Math.random());
double magv = circlev(px,py);
double absangle = Math.atan(Math.abs(py/px));
double thetav= Math.PI/2-absangle;
double phiv = Math.random()*Math.PI;
double vx = -1*Math.signum(py)*Math.cos(thetav)*magv;
double vy = Math.signum(px)*Math.sin(thetav)*magv;
//Orient a random 2D circular orbit
if (Math.random() <=.5) {
vx=-vx;
vy=-vy;
}
double mass = Math.random()*solarmass*10+1e20;
//Color the masses in green gradients by mass
int red = (int) Math.floor(mass*254/(solarmass*10+1e20));
int blue = (int) Math.floor(mass*254/(solarmass*10+1e20));
int green = 255;
Color color = new Color(red, green, blue);
bodies[i] = new Body(px, py, vx, vy, mass, color);
}
//Put the central mass in
bodies[0]= new Body(0,0,0,0,1e6*solarmass,Color.red);//put a heavy body in the center
}
//Use the method in Body to reset the forces, then add all the new forces
public void addforces(int N) {
for (int i = 0; i < N; i++) {
bodies[i].resetForce();
//Notice-2 loops-->N^2 complexity
for (int j = 0; j < N; j++) {
if (i != j) bodies[i].addForce(bodies[j]);
}
}
//Then, loop again and update the bodies using timestep dt
for (int i = 0; i < N; i++) {
bodies[i].update(1e11);
}
}
public static double exp(double lambda) {
return -Math.log(1 - Math.random()) / lambda;
}
public boolean action(Event e,Object o)
{
N = Integer.parseInt(t1.getText());
if (N>1000) {
t1.setText("1000");
N=1000;
}
startthebodies(N);
repaint();
return true;
}
public class ButtonListener implements ActionListener{
public void actionPerformed(ActionEvent evt)
{
// Get label of the button clicked in event passed in
String arg = evt.getActionCommand();
if (arg.equals("Restart"))
{
shouldrun=true;
N = Integer.parseInt(t1.getText());
if (N>1000) {
t1.setText("1000");
N=1000;
}
startthebodies(N);
repaint();
}
else if (arg.equals("Stop"))
stop();
}
}
}
And that's it! It's pretty simple once we have the Body object defined. This is easily embedded in a webpage using the
<applet code>
You can skip to the Applets page to see this one in action, or head to the Next section of programming, where we will look at the Barnes-Hut algorithm.
N-body Simulations- Code Part 3
Implementing the Barnes-Hut Algorithm: A Quadrant Class
In the Introduction we talked about an object called a Barnes-Hut tree. This is one of two additional parts to the Barnes-Hut algorithm. The other is the concept of a quadrant (in 2D) or an octant (in 3D). For coding simplicity, we will create a quadrant-based Barnes-Hut tree. It is not difficult to add additional nodes if need be.
To create a quadrant object, the code goes as follows (again, comments preceded by //):
//A quadrant object that can self-subdivide. Important for creating a Barnes-Hut tree, since it holds quadrants.
public class Quad {
private double xmid, ymid, length;
//Create a square quadrant with a given length and midpoints (xmid,ymid)
public Quad(double xmid, double ymid, double length) {
this.xmid=xmid;
this.ymid=ymid;
this.length=length;
}
//How long is this quadrant?
public double length() {
return length;
}
//Check if the current quadrant contains a point
public boolean contains(double xmid, double ymid) {
if (xmid<=this.xmid+this.length/2.0 && xmid>=this.xmid-this.length/2.0 && ymid<=this.ymid+this.length/2.0 && ymid>=this.ymid-this.length/2.0) {
return true;
}
else {
return false;
}
}
//Create subdivisions of the current quadrant
// Subdivision: Northwest quadrant
public Quad NW() {
Quad newquad = new Quad(this.xmid-this.length/4.0, this.ymid+this.length/4.0,this.length/2.0);
return newquad;
}
// Subdivision: Northeast quadrant
public Quad NE() {
Quad newquad = new Quad(this.xmid+this.length/4.0, this.ymid+this.length/4.0,this.length/2.0);
return newquad;
}
// Subdivision: Southwest quadrant
public Quad SW() {
Quad newquad = new Quad(this.xmid-this.length/4.0, this.ymid-this.length/4.0,this.length/2.0);
return newquad;
}
// Subdivision: Southeast quadrant
public Quad SE() {
Quad newquad = new Quad(this.xmid+this.length/4.0, this.ymid-this.length/4.0,this.length/2.0);
return newquad;
}
}
One more thing that is useful to update now is the Body class so we can check if the body is in a certain quadrant. To do this, all we have to do is add the following method, which takes advantage of the methods we just defined in the Quad class.
public boolean in(Quad q) {
return q.contains(this.rx,this.ry);
}
}
Now we have a data structure to hold Bodies, but what we really need is the Barnes-Hut tree that hold quadrants and contain information about the bodies they contain. The next section will do just that.
N-body Simulations- Code Part 4
Implementing the Barnes-Hut Algorithm: A Barnes-Hut Tree
Now, we need to create a Barnes-Hut tree that will hold the quadrants and bodies, and allow us to approximate far-away bodies by their total and center of mass.
The code is fairly complex and its methods are non-trivial, so it is necessary to really look through the code to see what is happening. The complexity draws for the recursive methods that are mentioned in the comments.
To create a Barnes-Hut tree, the code goes as follows (again, comments preceded by //):
import java.awt.Color;
public class BHTree {
private Body body; // body or aggregate body stored in this node
private Quad quad; // square region that the tree represents
private BHTree NW; // tree representing northwest quadrant
private BHTree NE; // tree representing northeast quadrant
private BHTree SW; // tree representing southwest quadrant
private BHTree SE; // tree representing southeast quadrant
//Create and initialize a new bhtree. Initially, all nodes are null and will be filled by recursion
//Each BHTree represents a quadrant and a body that represents all bodies inside the quadrant
public BHTree(Quad q) {
this.quad=q;
this.body=null;
this.NW=null;
this.NE=null;
this.SW=null;
this.SE=null;
}
//If all nodes of the BHTree are null, then the quadrant represents a single body and it is "external"
public Boolean isExternal(BHTree t) {
if (t.NW==null && t.NE==null && t.SW==null && t.SE==null) return true;
else return false;
}
//We have to populate the tree with bodies. We start at the current tree and recursively travel through the branches
public void insert(Body b) {
//If there's not a body there already, put the body there.
if (this.body==null) {
this.body=b;
}
//If there's already a body there, but it's not an external node
//combine the two bodies and figure out which quadrant of the
//tree it should be located in. Then recursively update the nodes below it.
else if (this.isExternal(this)==false) {
this.body=b.add(this.body,b);
Quad northwest = this.quad.NW();
if (b.in(northwest)) {
if (this.NW==null) {this.NW= new BHTree(northwest);}
NW.insert(b);
}
else {
Quad northeast = this.quad.NE();
if (b.in(northeast)) {
if (this.NE==null) {this.NE= new BHTree(northeast);}
NE.insert(b);
}
else {
Quad southeast = this.quad.SE();
if (b.in(southeast)) {
if (this.SE==null) {this.SE= new BHTree(southeast);}
SE.insert(b);
}
else {
Quad southwest = this.quad.SW();
if(this.SW==null) {this.SW= new BHTree(southwest);}
SW.insert(b);
}
}
}
}
//If the node is external and contains another body, create BHTrees
//where the bodies should go, update the node, and end
//(do not do anything recursively)
else if (this.isExternal(this)) {
Body c = this.body;
Quad northwest = this.quad.NW();
if (c.in(northwest)) {
if (this.NW==null) {this.NW= new BHTree(northwest);}
NW.insert(c);
}
else {
Quad northeast = this.quad.NE();
if (c.in(northeast)) {
if (this.NE==null) {this.NE= new BHTree(northeast);}
NE.insert(c);
}
else {
Quad southeast = this.quad.SE();
if (c.in(southeast)) {
if (this.SE==null) {this.SE= new BHTree(southeast);}
SE.insert(c);
}
else {
Quad southwest = this.quad.SW();
if(this.SW==null) {this.SW= new BHTree(southwest);}
SW.insert(c);
}
}
}
this.insert(b);
}
}
//Start at the main node of the tree. Then, recursively go each branch
//Until either we reach an external node or we reach a node that is sufficiently
//far away that the external nodes would not matter much.
public void updateForce(Body b) {
if (this.isExternal(this)) {
if (this.body!=b) b.addForce(this.body);
}
else if (this.quad.length()/(this.body.distanceTo(b))<2){
b.addForce(this.body);
}
else {
if (this.NW!=null) this.NW.updateForce(b);
if (this.SW!=null) this.SW.updateForce(b);
if (this.SE!=null) this.SE.updateForce(b);
if (this.NE!=null) this.NE.updateForce(b);
}
}
// convert to string representation for output
public String toString() {
if(NE != null || NW!=null || SW!=null || SE!=null) return "*" + body + "\n" + NW + NE + SW + SE;
else return " " + body + "\n";
}
}
Now these are all the data types we need to run the algorithm. It's obvious that they are much less trivial than the Body data type, but they are essential if we want to cut down run time. Next, we put it all together in our main class that actually runs the simulation.
N-body Simulations- Code Part 5
Implementing the Barnes-Hut Algorithm: Main Method
Now that we have all our objects described, it is fairly straight-forward to run the simulation. Below is the commented code--the function that has been changed is addforces(int N), which has been updated to use the Barnes-Hut method. Other than that difference, our object-oriented approach has allowed this code to look almost identical to the Brute Force case. One big difference is that now, instead of invoking a loop to add all the forces on a body, we now just call the updateForce(Body b) method from our BHTree class, which takes, on average, Log(N) time to run, versus a loop that would take N.
// tell the compiler where to find the methods you will use.
// required when you create an applet
import java.applet.*;
// required to paint on screen
import java.awt.*;
import java.awt.event.*;
//Start the applet and define a few necessary variables
public class BarnesHut extends Applet {
public int N = 100;
public Body bodies[]= new Body[10000];
public TextField t1;
public Label l1;
public Button b1;
public Button b2;
public boolean shouldrun=false;
Quad q = new Quad(0,0,2*1e18);
// The first time we call the applet, this function will start
public void init()
{
startthebodies(N);
t1=new TextField("100",5);
b2=new Button("Restart");
b1=new Button("Stop");
l1=new Label("Number of bodies:");
ButtonListener myButtonListener = new ButtonListener();
b1.addActionListener(myButtonListener);
b2.addActionListener(myButtonListener);
add(l1);
add(t1);
add(b2);
add(b1);
}
// This method gets called when the applet is terminated. It stops the code
public void stop()
{
shouldrun=false;
}
//Called by the applet initally. It can be executed again by calling repaint();
public void paint(Graphics g)
{
g.translate(250,250); //Originally the origin is in the top right. Put it in its normal place
//check if we stopped the applet, and if not, draw the particles where they are
if (shouldrun) {
for(int i=0; i<N; i++) {
g.setColor(bodies[i].color);
g.fillOval((int) Math.round(bodies[i].rx*250/1e18),(int) Math.round(bodies[i].ry*250/1e18),8,8);
}
//go through the Barnes-Hut algorithm (see the function below)
addforces(N);
//repeat
repaint();
}
}
//the bodies are initialized in circular orbits around the central mass.
//This is just some physics to do that
public static double circlev(double rx, double ry) {
double solarmass=1.98892e30;
double r2=Math.sqrt(rx*rx+ry*ry);
double numerator=(6.67e-11)*1e6*solarmass;
return Math.sqrt(numerator/r2);
}
//Initialize N bodies
public void startthebodies(int N) {
double radius = 1e18; // radius of universe
double solarmass=1.98892e30;
for (int i = 0; i < N; i++) {
double px = 1e18*exp(-1.8)*(.5-Math.random());
double py = 1e18*exp(-1.8)*(.5-Math.random());
double magv = circlev(px,py);
double absangle = Math.atan(Math.abs(py/px));
double thetav= Math.PI/2-absangle;
double phiv = Math.random()*Math.PI;
double vx = -1*Math.signum(py)*Math.cos(thetav)*magv;
double vy = Math.signum(px)*Math.sin(thetav)*magv;
//Orient a random 2D circular orbit
if (Math.random() <=.5) {
vx=-vx;
vy=-vy;
}
double mass = Math.random()*solarmass*10+1e20;
//Color a shade of blue based on mass
int red = (int) Math.floor(mass*254/(solarmass*10+1e20));
int blue = 255;
int green = (int) Math.floor(mass*254/(solarmass*10+1e20));
Color color = new Color(red, green, blue);
bodies[i] = new Body(px, py, vx, vy, mass, color);
}
bodies[0]= new Body(0,0,0,0,1e6*solarmass,Color.red);//put a heavy body in the center
}
//The BH algorithm: calculate the forces
public void addforces(int N) {
BHTree thetree = new BHTree(q);
// If the body is still on the screen, add it to the tree
for (int i = 0; i < N; i++) {
if (bodies[i].in(q)) thetree.insert(bodies[i]);
}
//Now, use out methods in BHTree to update the forces,
//traveling recursively through the tree
for (int i = 0; i < N; i++) {
bodies[i].resetForce();
if (bodies[i].in(q)) {
thetree.updateForce(bodies[i]);
//Calculate the new positions on a time step dt (1e11 here)
bodies[i].update(1e11);
}
}
}
//A function to return an exponential distribution for position
public static double exp(double lambda) {
return -Math.log(1 - Math.random()) / lambda;
}
public boolean action(Event e,Object o)
{
N = Integer.parseInt(t1.getText());
if (N>10000) {
t1.setText("10000");
N=10000;
}
startthebodies(N);
repaint();
return true;
}
public class ButtonListener implements ActionListener{
public void actionPerformed(ActionEvent evt)
{
// Get label of the button clicked in event passed in
String arg = evt.getActionCommand();
if (arg.equals("Restart"))
{
shouldrun=true;
N = Integer.parseInt(t1.getText());
if (N>10000) {
t1.setText("10000");
N=10000;
}
startthebodies(N);
repaint();
}
else if (arg.equals("Stop"))
stop();
}
}
}
And that's it! The objects were more complicated, but the end code is quite simple and elegant. To see both codes in action, go to the Applets page. Here, you can play around with N, the number of bodies, to watch the dynamics and get a sense for the how computationally intensive each method is.
Site Map
N-body Simulations